Chapter 4
PRACTITIONERS AND MECHANICIANS
The preceding two chapters have looked at the work of Thomas Digges and Mathew Baker, two important figures whose careers reveal markedly different aspects of the development of mathematical practice. Of the other Dover participants, several could also be made the object of chapter-length studies. However, the limited space available here does not permit the luxury of so extended an exposition. Therefore this chapter serves as a compendium, incorporating selective accounts of the various other figures who were involved at Dover and whose work was related to the mathematical arts.
The list of names is not long, but it is diverse: the navigator William Borough; Thomas Bedwell, a university graduate turned military engineer; Robert Stickells and John Symonds, who were both architects and working masons; Paul Ive, who acted principally as an expert on fortifications; and finally an auditor, John Hill. Examining their careers and practice opens up mathematical arts which were marginal in the previous chapters. These new subjects are considered in three sections on navigation and magnetism; mensuration and the strategic uses of mathematical instruments; and fortification, architecture and surveying. Though they are linked, [page 167:] and share my continuing concern with the identity of the mathematical practitioner, each of these three sections stands as a largely self-contained account.
1. NAVIGATION, MAGNETISM AND MATHEMATICAL PRACTICE
It has long been clear that navigation was a crucial discipline for the development of the mathematical arts in England.1 Between the mid-16th and the early 17th centuries there were dramatic changes in the procedures and instruments available to English navigators. Many of the most striking innovations were advocated by mathematicians or mathematical practitioners, and navigation became a key site for the exercise and display of mathematical expertise. Of course, it did not follow that novel techniques were adopted either widely or speedily. Mathematical procedures were primarily intended for problems of oceanic navigation, rather than for the familiar waters of coastal Britain or north west Europe. The ordinary mariner’s skills would long continue to rest on more general seamanship: the manning of rigging and steerage; the judgement of location by coastal profiles, by sounded depths and the nature of the sea bottom; the estimation of tides; and the knowledge of local dangers in harbours and rivers. But despite such major continuities, the printed word was the medium for new techniques, which accordingly received the lion’s share of public attention and debate.
The pre-existence of well-established maritime traditions meant that the [page 168:] navigational work of mathematical practitioners was never carried out in a vacuum, or on a specialised intellectual territory entirely their own. There were always interested parties who could and would comment on the practitioners’ public conclusions or recommendations. Moreover, the juxtaposition with existing practice raised questions about the attribution of authority in navigation. Whose pronouncements carried greatest weight and what skills were most central to navigational expertise? Was it an ability in spherical trigonometry coupled with facility in the observational techniques appropriate to mathematical instruments? Or was actual experience of voyages and the conditions of shipboard life of greater importance? Claims about the relative merits of reason and experience became commonplace in navigational debate. In this section I want to decipher what was at stake in such debates, for the resolution of particular questions of navigational expertise had more general consequences for the role of the mathematical practitioner. Indeed, deliberations on navigational mathematics had implications not just for the proper personnel and practice of navigation but also for other mathematical arts.
The major character in my discussion of navigation (and especially navigational magnetism) is William Borough. However I want to begin not with Borough but with Thomas Digges. Digges, whose career has already been discussed in chapter 2, began publishing in the early 1570s with texts on self-consciously novel and advanced areas of the mathematical sciences. Later in his career he reoriented his priorities and increasingly advocated and practised mathematics for its civic and military utility. He shifted his intellectual work from that of a learned mathematician to a mathematical practitioner, helping to publicly define that role in England. Although never fulfilling his repeated promises to publish a treatise on the subject, his limited navigational output nevertheless reveals the significance of this area for his [page 169:] changing identity as a mathematician.
Digges’s principal published text on navigation dates from 1576, though he had by then already shown an awareness of navigational problems: in 1573 he remarked that some of his recommendations for the radius astronomicus could also be applied to the mariners’ cross staff.2 His text of 1576 is part of the same addition to Leonard Digges’s Prognostication Everlasting that contained the much-better known ‘Perfit Description of the Celestial Orbs’.
The navigational section of Digges’s addition consisted of two parts: ‘A short discourse touching the variation of the compass’ and ‘Errors in the Art of Navigation commonly practised’. The discussion of navigational errors is presented in a straightforward manner. Digges briefly diagnosed five principal faults in contemporary practice, listing problems with the construction and use of charts and instruments, as well as pointing to both empirical and geometric flaws in standard procedures. He also lamented the lack of a secure method of establishing longitude, the ‘one great imperfection yet in their art.’
For reformation of these errors and imperfections, new charts, new instruments and new rules must be prescribed. Wherein I have prepared in a peculiar volume for that purpose to entreat, wishing in the meantime that such as are not able to reform these faults will abstain to teach our countrymen more errors (sig. P2r).
Digges clearly cast himself as a corrector of these manifold errors, able to hand down improvements to navigators. That his convictions rested on an evaluation of the importance and power of mathematics becomes clear from his treatment of variation. [page 170:] Although occupying only 3½ pages, Digges’s discussion is a rich source for his views on the respective responsibilities of mariners and mathematicians.
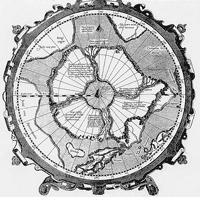
Variation is the angle between a magnetic needle’s indication of direction and the geographical meridian. From hydrographical evidence, it seems to have first been discovered late in the 14th century by Portuguese pilots.3 But this troubling phenomenon, which could confound an oceanic navigator’s reliance on the compass as a trustworthy indicator of direction, was not widely recognised until printed discussions appeared in the 16th century. In reviewing contemporary opinions on the cause of the variation, Digges briskly dismissed the majority as absurd. The most probable and widely canvassed of current theories was the notion of an attractive point to which all needles would point. The terrestrial version of this ‘polar model’ of variation was most graphically displayed on Mercator’s 1569 world chart, which showed an enormous magnetic rock some 16° from the geographical north pole (Figure 4.1). But Digges rejected even this most plausible of explanatory candidates: after first establishing the position of the magnetic pole from observations at two locations, a third observation of the needle at a fresh geographical position would typically show the needle pointing elsewhere.
Digges therefore tentatively offered one of his own suggestions as ‘an hypothesis or supposed cause of the variation of the compass’. But he made it aggressively clear that this offering was only to be assessed by a restricted audience of mathematically competent judges. It was ‘to be considered, weighed and examined by exquisite trial of geometrical demonstration and arithmetical calculation, for it is no question for gross mariners to meddle with, no more than the finding of the [page 171: Figure 4.1] [page 172:] longitude’ (sig. O4r). Digges made it painfully clear that he considered even the most renowned navigators of the age to fall within the class of ‘gross mariners’.
I cannot a little wonder at the blind boldness of Sebastian Cabot and some others that being ignorant both in geometrical demonstration and arithmetical sinical calculations have nevertheless taken upon them[selves] in these most difficult questions to promise resolution, being no more able or likely to perform it than an ox to fly between two mountain tops (sig. O4r).4
Digges conceded that such navigators did indeed deserve praise for their voyages and discoveries, but he recommended that they desist from intellectual claims: ‘let them learn Apelles’s lesson ne sutor ultra crepidam’. Mariners were no more than mechanicians whose ambitions should not overstep their limited abilities; only the mathematically expert could tackle the truly fundamental questions of navigation.
Digges’s radical attribution of navigational competence was matched by the form of his variation hypothesis, for he ingeniously attributed the global pattern of variation to little more than geometry. He suggested that all magnetic needles have the same preferred orientation with respect to the earth, independent of their geographical position (thus he rejected the notion that needles naturally point to a single pole). However, magnetic needles also have a second constraint: at any given latitude and longitude they must balance in their local horizontal plane. (Digges evidently did not know of Robert Norman’s discovery, published five years later, that needles dip below the horizon.) In Digges’s hypothesis, a needle will therefore come to rest in the line defined by the intersection of the magnetic and horizontal planes. This is sufficient basis for a geometric model in which, after the disposition of the [page 173:] earth’s parallel magnetic planes has been found (in effect, a prime magnetic meridian established), variation can be readily calculated given local latitude and longitude.5
However, Digges’s verbal presentation was brief, not to say obscure. He gave no diagrams or numerical examples to aid understanding. His minimalist expository strategy confirms that the account was aimed only at those already instructed in the mathematical arts. Indeed, his only didactic concession was to offer an analogy between magnetic variation and the layout of sundials which both decline and incline. The analogy was intellectually and socially exclusive: it offered illumination only to those with prior expertise in the mathematical art of dialling.6
No observations were offered to bolster Digges’s exposition. But he did not apologise for the absence of empirical support; on the contrary, the unavailability of reliable evidence was a further indictment of the inadequacies of seamen.
Of the verity of this supposition I could easily determine if there were any trust to the observations of mariners, but having found by experience their gross usage and homely instruments, where [page 174:] half a point commonly breaks no square, and also their repugnant tales that have travelled the self same voyage, I cannot yet resolve (sigs O4v-P1r).
Digges expressed no embarrassment at his highly theoretical position and exhibited no defensiveness over the lack of connection between his hypothesis and the realities of navigational practice.7 His intemperate dismissal of existing navigational skills can scarcely have endeared him to seafaring contemporaries. Nor did his first attempts at personal persuasion and intellectual conversion meet with any immediate success. Writing retrospectively in 1579, he noted that
by masters, pilots and mariners I have been answered that my demonstrations were pretty devices, but if I had been in any sea services I should find all these my inventions mere toys and their rules only practicable, adding further that whatsoever I could in paper by demonstrations persuade, by experience on seas they found their charts and instruments true and infallible.8
Digges continued this train of retrospective reflection by recounting the doubts he had experienced in the face of these mariners’ vehement rebuttals. He admitted that his confidence in mathematical demonstrations had been weakened by the mariners’ unswerving defence of their own practices. But Digges went on to triumphantly announce his subsequent vindication when, after spending 15 weeks at sea, ‘by proof I found, and those very masters themselves could not but confess, that experience did no less plainly discover the errors of their rules, than [did] my demonstrations’. Thus Digges’s voyage was presented as a victory for the paper work of a landsman’s mathematical reason, against the seaman’s vulgar experience, highlighting what Digges took to be the clear superiority of his own skills. [page 175:]
Unfortunately, we have only Digges’s side of the story. What would his mariner companions have said? They might have pointed out Digges’s need to abandon his armchair and personally go to sea in order to convince himself of his own supposedly demonstrative conclusions. But we are not restricted to mere speculation on the range of responses encountered by Digges, for his attempted subjection of mariners to mathematical expertise was itself shortly to become the object of public criticism.
Robert Norman, mariner turned compass-maker and hydrographer, published his little book The New Attractive in 1581. While touching on matters such as the variation of the compass and the practical techniques of navigation, the central purpose of Norman’s text was to announce his discovery of the phenomenon of magnetic dip (the deviation from horizontal of a magnetic needle free to rotate in a vertical plane). Among the many remarkable features of this book is the clear rebuke which Norman gave to Digges in his preface to the reader. Digges is not actually named but, to anyone familiar with his 1576 text, there could be no doubt that he was the object of Norman’s censure. Compare the following quote from Norman with Digges’s own words cited above (p. 170),
it may be said by the learned in the mathematicalls, as hath been already written by some, that this is no question or matter for a mechanician or mariner to meddle with, no more than is the finding of the longitude, for that it must be handled exquisitely by geometrical demonstration and arithmetical calculation, in which arts they would have all mechanicians and seamen to be ignorant, or at least insufficiently furnished to perform such a matter, alleging against them the Latin proverb of Apelles, Ne sutor ultra crepidam (sig. B1r-v).
However, Norman’s criticism of Digges was primarily social rather than [page 176:] technical. He did not dispute the validity of Digges’s listing of navigational errors or argue against his hypothesis of variation. Rather, he contended that mechanicians not only have the right but many also have the ability to make their own intellectual and empirical contributions. Learned authors such as Digges, ‘being in their studies amongst their books can imagine great matters and set down their conceipts in fair show and with plausible words’, but they should not expect to parasitically exploit their more humble compatriots.9 According to Norman, the learned wished ‘that all mechanicians were such as for want of utterance should be forced to deliver unto them their knowledge and conceipts, that they might flourish upon them and apply them at their pleasures’.
Norman was rejecting Digges’s crude demarcation between expert mathematicians and gross mariners. He asserted that, through the availability of vernacular mathematical texts, mechanicians already had the means for sufficient instruction in geometry and arithmetic. The New Attractive was intended to demonstrate that the mechanician need not be an inarticulate drudge but could legitimately participate in both the pleasures of discovery and the publication of results.10
Robert Norman was not among the ranks of the Dover practitioners. Nevertheless, his work is essential if we are to appreciate the depth of response provoked by Digges’s navigational intervention. Here was a mere mechanician taking it on himself to publicly reprove a man whose social station was far above his own. [page 177:] Digges was a substantial esquire with increasingly important political connections. Within the elaborately hierarchical society of Elizabethan England, Norman’s action required extraordinary temerity. Little wonder that Digges was not mentioned by name.
Moreover, though Norman did not work at Dover, one of his closest associates did. The New Attractive was dedicated to William Borough, who was described as the prime stimulus to Norman’s discovery of magnetic dip. The extent of their cooperation is attested by the circumstances of publication: Norman’s book did not appear alone but, bound with it, was Borough’s only publication, A Discourse of the Variation of the Compass or Magnetical Needle. I want to argue that Borough’s slim booklet (along with his other navigational and hydrographical work) negotiated a working compromise between the imperious demands of Digges the mathematician and the sharp rejoinder of Norman the mechanician. Borough sought to settle the evident differences over who was competent to speak on navigational matters. Although his work was principally intended to exemplify the proper credentials for a student of navigation, it also implied the more general requirements appropriate to the role of the mathematical practitioner, a role whose rhetorical and practical programme was interpreted to exclude neither the learned nor the humble.
Before considering Borough’s work, a brief review of his career is in order. Borough was brought up in a seafaring family. Although definitive confirmation is lacking, his father was almost certainly John Aborough, one of the first Englishmen to equip himself with the Iberian-developed tools of oceanic navigation.11 William’s [page 178:] elder brother was the navigator Stephen Borough who, during the reign of Philip and Mary in the 1550s, was invited to the Casa de la Contratación in Seville. He was later appointed as one of the four masters of the Queen’s ships in the Medway.12
Given this family background it is scarcely surprising that the first reference to William Borough is as a youthful mariner: in 1553, aged sixteen, he was serving under his brother on the first English voyage in search of the north east passage. Although no route was found to the fabled riches of the east, this voyage laid the foundations for the Muscovy Company’s trade with Russia, and William quickly became one of the Company’s principal navigating masters. He served the Muscovy Company almost continuously for some 25 years, making regular voyages to the White Sea and the Gulf of Finland and acting variously as pilot, admiral and Company agent.13
After rising to prominence in these trading voyages, Borough transferred his services to the crown, occupying a sequence of administrative positions on the Navy Board from the beginning of the 1580s onwards. Respected for his navigational expertise, Borough also offered advice on various other technical matters such as the [page 179:] design of ships.14 His participation in the Dover harbour works fits neatly into the widening circle of his career. Moreover, as well as serving as a reliable administrator and consultant, Borough was also called on as a commander, and he often acted as an admiral or vice-admiral in royal warships.15 Wider recognition of his abilities was forthcoming in 1585 when he was appointed Master of Trinity House (a position that Stephen Borough had also held).16 At the time of his death in 1598, Borough could look back on a career exemplary for its upward mobility. He was Controller of the Navy, was entitled to bear arms and had taken the Lady Jane Wentworth as his second wife. He had also become extremely wealthy: in his will he made provision for payments of up to £3000 in ready money.17
But Borough was more than just a successful navigator making his way into the Elizabethan high establishment. His publication of the 1581 Discourse made clear his intellectual commitment to the mathematical arts, and this text was only the most visible index of his long-term endeavours in navigation and hydrography.
Borough’s concern to establish the proper character of the navigator and map maker is first evident in a 1578 letter to the queen. The letter was written as a dedication to accompany a (now lost) manuscript map of Russia and its coasts which Borough had drawn up on the basis of his extensive travels in the region.18 Borough [page 180:] emphasised that he had used his own careful notes and observations as the basis for the map, only drawing on other cartographic work (principally Mercator and Ortelius) for those areas that he had not himself travelled through. The stress on personal observation was not a simple advocacy of practical experience at the expense of scholars who remained confined to their bookish studies. Borough stated that not only were navigation and hydrography mutually interdependent but that both required the assistance of astronomy and cosmography, not to mention the even more fundamental help of geometry and arithmetic, ‘these two grounds of all arts’. In short, he swore allegiance to the programme of the mathematical arts.
But Borough could scarcely accept Digges’s polarisation of the learned mathematician and the gross mariner; to do so would be to betray his own upbringing and profession. Borough opted to characterise mathematical learning as necessary but not sufficient in matters of navigation:
none of the best learned in those sciences mathematical, without convenient practice at the sea, can make just proof of the profit in them; so necessarily dependeth art and reason upon practice and experience.19
He complained of those inexperienced pundits who ‘in talk of navigation will enter deeply and speak much of and against errors used therein when they cannot reform them’. Borough perhaps had Digges’s recent 1576 text in mind when he took up the challenge of those who had published on navigational errors.
It is so, that there are rules used in navigation which are not perfectly true, among which the straight lines in sea cards, representing the 32 points of the compass or winds, are not holden to be the least, but noted of such talkers for principal, to condemn the occupiers thereof for ignorant: yet hath the famous and learned Gerard Mercator used them in his universal map. But such as condemn them for false, and speak most against their use cannot [page 181:] give other that should serve for navigation to better purpose and effect (ibid.).
If Digges was his target here (and the error of straight-lined rhumbs was second in his list), then Borough scored a palpable hit. For, despite referring to his own navigational treatise in 1576, Digges never did deliver the new rules, instruments and charts that he promised.20
Borough developed his rhetorical prescription for the combination of reason and experience more fully in his Discourse of 1581. The prescription was implemented technically into a range of procedures for the determination of variation. It was also implemented socially, for this range of procedures was intended to make Borough’s text accessible to the maximum number of his countrymen. He proceeded ‘both practically and mathematically, to the end I might partly satisfy both the vulgar and also the learned sort’ (sig. *2r). How did Borough set about reconciling the divergent expectations and simmering antagonisms of the navigationally engaged public?
The Discourse opens with definitions and a discussion of the variation instrument commercially available at Robert Norman’s house at Ratcliffe. (Borough also added his own newly improved variation instrument at the end of the book). There then follows a series of chapters on different methods of determining variation. These chapters are arranged in order of mathematical sophistication, from the equal altitude observational method, through the use of the globe as a conceptual and [page 182:] computational aid, to techniques which demanded competence in the spherical trigonometry more typically associated with mathematical astronomy.
Borough recommended that his readers follow whichever methods best suited their interests and abilities (sig. *2v). For an ordinary mariner, the method of observing the sun at equal altitudes in both the morning and afternoon involved no more mathematical sufficiency than addition and subtraction. Equally, the interests of the expert mathematician were accommodated, for Borough was very far from ignorant in the ‘geometrical demonstration and arithmetical sinical calculations’ that Digges had dictated as essential. Borough was comfortable with the Latin mathematics of Regiomontanus, Copernicus, Rheticus and Rheinhold. Indeed, the more advanced aspects of Borough’s presentation may have served not just as an exposition of mathematical procedures but also as a means of heading off the possibility of mathematical attack. Certainly, these chapters were later viewed rather as ingenious exercises than practical techniques, even in a learned text such as William Gilbert’s De Magnete.21
Borough thus endeavoured to draw in all the relevant constituencies to whom appeal could be made. But it is clear that his sympathies lay more with those who practised than studied. Just as Digges had enumerated errors in the contemporary practice of mariners, so Borough conversely took a certain pugnacious pleasure in highlighting difficulties with the texts and maps of noted continental authorities. Even [page 183:] though he otherwise admired their learning, Borough found faults with particular points in Petrus Nonius and Gerard Mercator, while more sweeping criticism was reserved for Guillaume Postel, Michiel Coignet, and Pedro de Medina, the latter of whom ‘reasoneth very clerkly’.22 Nevertheless, Borough studiously sought to avoid a charge of partisan behaviour, for he did not spare his seafaring colleagues; he identified the neglect of variation as a prime cause of confusion in contemporary chart-making, a problem compounded by the different European traditions of compass manufacture (in which the compass needle was offset under the fly to adjust for different local variations).23
Borough was also able to demonstrate the rewards of mathematical skill. Norman’s and Borough’s texts are generally in agreement, as we would expect from their acknowledged cooperation. But there is one point on which they differ. In his seventh chapter, Norman says that, on the basis of his single determination of magnetic dip at London, it is not possible to establish to what point inside the earth the needle points. Borough showed not only that this point could be mathematically found but he carried out the calculation, assuming no more than the standard location for the magnetic meridian.24
The Discourse thus threaded its way through a minefield of potential controversy. One of the most testing obstacles to overcome was the global behaviour of variation, as opposed to just its local determination. Digges had offered his [page 184:] geometric model, divorced from the untrustworthy observation reports of vulgar mariners. But Borough was able to confront existing models with his own observations. Unfortunately, he did not discuss Digges’s hypothesis but reserved his attention for the polar model of variation. Digges had dismissed in just a few words the conception of a single magnetic pole to which all needles would point, stating simply that it was contrary to the evidence. Borough came to the same conclusion, but rested his case not on authoritative rejection but on a full exposition.
In chapter 8, he detailed the spherical trigonometry required to determine the position of the supposed magnetic pole, using as data the acknowledged magnetic meridian passing by the Azores and his own determination of variation at London as 11¼° East. In chapter 12 he returned to the topic to spell out some of the principal features of variation behaviour according to the polar model. Then he compared the model’s theoretical prediction of variation with an observation of his own. He chose the remote island of Vaigatz (at the southern tip of Novaya Zemlaya in 70° latitude) where he had himself been and for which he thus had values of latitude, longitude and variation which he trusted. The model predicted a variation value of 49° 22’ East; Borough had determined it observationally as 7° West.25 This enormous discrepancy was not presented as an isolated crucial experiment, for ‘the like effect I have found by diverse observations in sundry other places of the east parts.’ Moreover, using Mercator’s 1569 universal map, Borough was able to ‘reverse engineer’ the results of [page 185:] Mercator’s observation of variation at Ratisbon (Regensburg). He found this to be less than the theoretical prediction, ‘which confirmeth the retrograde quality in the variation from hence eastwards’.
Though Borough rejected the polar model, he did not abandon all hope of finding a mathematical model which could accommodate the variation’s observed behaviour. He hoped ‘(if it be possible) to find some hypothesis for the salving of this apparent confused irregularity’.26 But to reduce the variation to order required a better representation of its geographical pattern, and this in turn required more observations. Borough accepted that he could not personally gather the wealth of requisite evidence and he had therefore already begun to commission reports from other travellers. He referred to the many observations that ‘I have caused to be made and daily procure to be done in diverse other countries’.27
But there was a grave problem with this programme of collecting observations: how to ensure the fidelity and reliability of the observers? Borough may [page 186:] not have been sympathetic to Digges’s condemnation of mariners’ ‘gross usage and homely instruments’ but he accepted that there was a serious issue at stake. Indeed, on the related topic of collating cartographic information provided by travellers, he was well aware of the scope for problems. He criticised Spanish and Portuguese charts, attributing their errors to the Iberian system of production in which chartmakers did not themselves gather observations but were supplied information by those who did. Thus, no matter how expert were the land-based chartmakers they were forever dependent on the reports of others. And Borough had little confidence in the ‘unskilful mariners’ of Iberia, who made no provision to systematically record observations during their voyages.28 The moral drawn from this state of affairs by Borough was that quality could only be assured by adequately instructing observers and providing them with suitable instruments. This was not a mere policy recommendation but a conclusion that he vigorously prosecuted as an adviser to various English exploratory voyages: several sets of his written instructions governing instruments, observations and information procedures survive.29
Borough wanted his observers to be reliable intermediaries, transparently reproducing their instructor’s own techniques. But his motives in thus attempting to [page 187:] ‘act at a distance’ were open to misinterpretation. There is a fascinating though obscure passage in the diary of Richard Madox which suggests that Borough’s efforts to accumulate evidence could be uncharitably construed. Madox was chaplain on Edward Fenton’s 1582 voyage and in his diary he recorded that
Vice-admiral [Luke] Ward makes many inquiries of me concerning the orderly movement of the stars and reports that William Borows is one who could fill his honeycombs with someone else’s honey and so far he is accustomed to feed French dogs while they bring in the hares. Thereupon he [Ward] sneers at such men and thus he admonishes me with promises and bland courtesy when I return home not to impart more than enough to him who, fully informed concerning all things, like a mule seeks his mother with his heels. For thus he made use of a certain learned and noble Scot.30
While the allusions may be cryptic, Ward’s disdain for Borough is not. Borough was attempting to discipline observers to follow his procedures and to provide him with reports for centralised collation and processing. But Ward saw Borough as a plagiarist, ungratefully appropriating others’ work. Ironically, the terms of his criticism recall Robert Norman’s trenchant critique of learned mathematicians and their desire to commandeer mechanicians’ results. Yet from my account it is apparent that Borough pursued a rather different strategy from that advocated by Digges in the 1570s. Convinced of the value of the mathematical sciences for the pursuit of navigation and hydrography, Borough nevertheless did not spurn his unlearned colleagues. Indeed, he encouraged them to study arithmetic and geometry and generalised the benefits of such study beyond the particular case of navigation. Furnished with the two basic mathematical arts, the seaman or traveller
may not only judge of instruments, rules and precepts given by other but also be able to correct them and to devise new of [page 188:] himself. And this not only in navigation but in all mechanical sciences (sig. *3v).
Borough’s work thus implied a vision not just of the role of the navigator but of the mathematical practitioner in general. It was a vision in which Digges’s sharp social distinctions were dismantled. That it became accepted as an appropriate formula for mathematical practitioners is most strongly suggested by the evidence of Digges himself.
Late in his career, Digges published second editions of both Pantometria and Stratioticos. One small addition to the former neatly encapsulates the transformation in outlook implied by his post-1570s identity (see above p. 168 and, for more on this transformation, chapter 2). The first edition of Pantometria contained a short ‘note for sea cards’ promising a treatise on cosmography and navigation. The expanded note of 1591 still promised this future treatise but now added that it would enable
the skilful and more learned sort of mariners [to] understand how to make such kind of observations of the variation of their compass in their Indian navigations, especially in circulating and environing the earth, as may reduce that most strange and irregular alteration of the nautical compass to a theoric certain (p. 50).
This passage could almost have been written by Borough! Gone was Digges’s earlier sweeping characterisation of gross mariners and his previous proposal for a purely geometric model of variation. Digges now allowed that there existed expert mariners whose observations would provide key data in establishing a ‘theoric’ of terrestrial magnetism to match those devised by astronomers for the heavens.
Younger mathematicians interested in navigation understood the message implicit in Digges’s changing navigational pronouncements and his spell at sea. One need only recall the examples of Thomas Harriot and Edward Wright, both of whom [page 189:] learnt the hard realities of observation on oceanic voyages.31 Their careers show that even the most advanced mathematicians of the next generation were closer to Borough’s prescription for the practitioner than to Digges’s recommendations of the 1570s. Navigation (and particularly navigational magnetism) had become a crucial location for the establishment of the mathematical practitioner’s role.32
2. THE USES OF MATHEMATICAL INSTRUMENTS
As with navigation, so instruments are of uncontested importance in the development of the mathematical arts in England. The Dover practitioners are exemplary in this respect. We have already considered Thomas Digges’s radius astronomicus and his publication of the surveying instruments described by Leonard Digges in Pantometria. Likewise, paper instruments for proportional calculations constitute a large part of Mathew Baker’s shipbuilding manuscript while, as well as devising a new instrument for magnetic variation, William Borough also made recommendations for the use of specific survey instruments. Instruments were thus as vital and tangible an element of the material culture of mathematical practice as were the texts in which they were described.33 Moreover, just as published texts [page 190:] physically emerged from the small world of the London printing houses, many practitioners’ instruments were manufactured in the workshops of the first commercial makers: the foundation of instrument making as a London-centred trade precisely coincided with the practitioners’ advocacy of their own new and improved devices.34
In this section I want to look at the uses of mathematical instruments; not so much the uses for measurement and calculation that were described in texts, but their utility in the establishment of individual careers and the creation of a social space for the practitioners. To do so, I will take the case of Thomas Bedwell, one of the Dover practitioners who has not yet been considered in detail. Bedwell’s career reveals particularly clearly how instruments were used as both material and symbolic tokens in relations between practitioners, patrons and mechanicians.
Bedwell first appears at Cambridge, entering university in 1562 and progressing to a fellowship at Trinity College.35 Apart from belonging to the small minority of matriculants who completed the full arts curriculum, he appears neither distinguished nor unusual. He could easily be classed as a student seeking a standard career such as preferment into the church. But Bedwell went in a very different direction. After leaving Trinity in about 1574 he is not heard of again until 1582, [page 191:] when he became a technical consultant and supervisor at Dover harbour.36 Bedwell’s involvement with the Dover project meant that he was no longer moving in academic circles but among Privy Councillors, gentlemen, craftsmen and labourers. For much of his subsequent career Bedwell continued to serve in the same environment, working particularly on military construction projects.
During his participation in the Dover works, Bedwell was referred to as ‘my Lord Chamberlain’s man’, placing him as a client of Thomas Radcliffe, 3rd Earl of Sussex.37 Sussex died in 1583 and Bedwell is next found in late 1584 serving the Earl of Leicester as a supervisor of works at Wanstead House.38 At the end of the following year, he was part of Leicester’s expeditionary force against the Spanish in the Netherlands, where he acted as colonel of the pioneers, directing the unarmed men who built ramparts, threw up earthworks and dug trenches.39 By the time of his return to England his reputation had spread more widely and he was in demand for tasks such as the surveying of fortifications.40 During the Armada crisis of 1588 he was again pressed into service, co-operating with the Italian engineer Federico Genebelli on strengthening the defences of the River Thames.41 After this sequence of separate projects he successfully petitioned in 1589 for a permanent position as [page 192:] Keeper of the Ordnance Store at the Tower of London, an office which he held until his death in 1595.42
Given the episodic character of much of his career, Bedwell depended on the favour of those nobles and administrators who controlled the distribution of offices and rewards. Instruments were part of his claim to credit and credibility. At the same time, he sought to differentiate his own status from the mechanicians and labourers whose work he supervised. Instruments were again assigned a role in these efforts. For Bedwell, the inventive ability required to devise instruments (as well as the expertise needed to understand their operation) justified the practitioners’ elevation above the ranks of artisans.
In the course of his career, Thomas Bedwell designed two instruments and wrote a pair of accompanying tracts to explain their respective uses. The first, a carpenter’s rule, was conceived early in his career and, although not widely known in Bedwell’s own lifetime, was publicised in the seventeenth century by his nephew, the Arabic scholar and mathematical author William Bedwell (Figure 4.2).43 Some years after devising his carpenter’s rule, Thomas created another instrument, similar in principle and appearance to its predecessor, but for the quite different purpose of gunnery.44 These two rules, tailored to the practical arts of mensuration and artillery, place Bedwell firmly within the contemporary mathematical arts. That Bedwell was self-consciously aware of his role as a mathematical practitioner is [page 193: Figure 4.2] [page 194:] evident from his retrospective reflection on the time when he first applied himself to the ‘profession of the mathematicalls’.45
However, Bedwell’s career poses us with a problem. For there was no obvious connection between the opportunities and expectations of a young scholar of the 1570s and the world of construction and military engineering which Bedwell entered in the 1580s. How did he make the transition between these two spheres? Bedwell lacked the training and experience of the typical military engineer, who had either learnt his trade as a craftsman (a mason, for example), or had picked it up as a military gentleman exercised in the art of war.46 He therefore needed to establish his credibility by presenting an alternative set of qualifications, which could persuasively demonstrate his expertise. His need to bridge a perceived credibility gap would have been particularly acute at the outset of his career, when he was least experienced. To establish the grounds on which Bedwell based his claims to technical competence we should therefore examine his earliest documented efforts to seek employment at Dover harbour.
In April 1582, Bedwell submitted a memorandum to Lord Treasurer Burghley in which he set out the credentials which he hoped would capture the Lord Treasurer’s attention and favour.47 The memorandum began with a note of some devices and techniques which could be profitably employed in the harbour works at Dover. However, Bedwell’s submission was not restricted to the particular matter in hand, for he appended an additional three points to his document. These points were [page 195:] evidently intended to show the breadth of his concerns: he offered to make a water clock to find the longitude during oceanic navigations; he expected to successfully conclude ballistic investigations into the ‘course of the bullet of great ordnance’; and he had devised an instrument for measuring timber and stone, ‘the easiest and most perfect that hath been or can be invented’. Each of these points - longitude, artillery and mensuration - fell within the domain of ‘the mathematicalls’. Although nothing more is known of the water clock, the measuring instrument is his carpenter’s rule and the concern with ordnance presages the development of his later gunner’s rule. It was thus through practical mathematics that Bedwell sought to convince Burghley of his suitability for employment in the harbour works. Indeed, his use of the water clock and carpenter’s rule indicate that Bedwell gambled on mathematical instruments as the most impressive way to convince the Lord Treasurer of his merits. Bedwell’s subsequent participation in the harbour works is evidence of the success of his persuasive strategy and suggests that the carpenter’s rule was a significant asset in achieving preferment and patronage, and in the founding of a novel career.
Bedwell’s use of instruments to procure patronage was not limited to his engagement in the Dover works. At the end of the 1580s he repeated the tactic when he sought out a permanent position to pursue and complete his studies of ordnance. The Dover memorandum indicates that Bedwell was already occupied with artillery problems in 1582. But it was not until about five years later, after returning from the Netherlands, that he designed his gunner’s rule.48 The device was to have a twin purpose: it could be used both for the correct elevation of artillery pieces and also for determining the range of fire for any given elevation. The engraving of scales for elevation was a problem of geometry and calculation, and gave the rule an outward [page 196:] appearance similar to the earlier carpenter’s rule. However, Bedwell’s second purpose, to make the rule serve as a ready reckoner for artillery ranges, posed a more taxing problem. A practical solution to the problem of predicting range was being sought throughout Europe at this time. For example, Niccolò Tartaglia had promised to publish tables giving just this data, but his promise went unredeemed.49 Bedwell was thus broaching one of the major unresolved technical issues of the day and his procedure is of considerable interest.
From his account, it would seem that Bedwell began by assuming a particular form for the projectile’s trajectory and that on this basis he then calculated theoretical range results. But he was dissatisfied with a purely theoretical procedure. Bedwell wanted some way of verifying or refining his results and he therefore planned to carry out a systematic series of experimental artillery trials. However, his limited means as a private individual did not stretch to so expensive an undertaking: in order to see his investigations through, he needed access to powder, shot and artillery. Bedwell’s appointment as Keeper of the Ordnance Store at the Tower of London in early 1589 placed him in direct control of the national stocks of exactly those resources which he required.50 Furthermore, even without Bedwell’s intervention, the artillery did not lie undisturbed in store but was regularly used for training purposes.51
How did Bedwell secure this post, so finely tuned to his explicitly stated needs? As at Dover, Bedwell’s appointment was effectively granted by Lord [page 197:] Burghley, but in this case Bedwell was able to press his suit through an appropriate intermediary, the Earl of Warwick, Master of the Ordnance.52 The arguments in his tract on the gunner’s rule show that Bedwell used the instrument in his bid for preferment. Claiming that a programme of experimental trials would give him a test of his otherwise uncertain rule, he held out the prospect of an exact instrument. Moreover, he declared that he would be able to determine the ‘perfect protract of the bullet’s range in the air, which is very corruptly imagined, supposed and described by all that have written thereof hitherto’.53
These inducements helped Bedwell to secure his permanent position as storekeeper. At both Dover and the Tower he obtained office and employment by using one of his own mathematical instruments to capture the favourable interest of noble patrons; mathematical instruments were crucial to the building of his career.
However, Bedwell’s instruments were not implicated only in his patronage relations, for he envisaged their primary users to be mechanicians. Although ostensibly devised to help the mechanician, instruments actually played a more subtle role in opening up a social space for the mathematical practitioner. Bedwell considered that, as users, humble mechanicians would have a double dependence on the mathematical practitioner: the practitioner would not only provide the expertise which mechanicians lacked but would also act as an intermediary between [page 198:] mechanicians and their social superiors. In his engineering work, Bedwell became accustomed to leading and directing the work of labourers and artificers; when discussing the uses of his instruments he implicitly justified this superior position. The instruments themselves provided a material embodiment of his claims to recognition and status.
Bedwell sought to accomplish his displacement and demotion of mechanicians by a two-step process. Firstly he emphasised the manifold errors common in daily practice. Then he offered his instruments as reliable replacements for the current devices and procedures. The strategy was in essence a simple one: having highlighted existing disorders and abuses the mathematical practitioner could step in to offer ready means for their reformation.
This tactic was employed with both the carpenter’s and gunner’s rules. When writing on the carpenter’s rule Bedwell gave an example of an artificer who, given a measure of breadth, will desire to find out what length of the material is needed to make up a square foot. But, ‘because they know not how to do it truly or at least not readily, my purpose is by this instrument to show them how to perform the same with reasonable exquisiteness and marvellous speed and celerity’.54 In the case of the gunner’s rule Bedwell sketched a similar situation. Gunners measured the elevation of their pieces of great ordnance in inches and had a special form of ruler for this task. It seemed to Bedwell that this procedure bred confusion and complexity. For, since each piece of ordnance was typically of a different length, mounting different pieces to the same elevation meant that each piece had to be raised to a different number of inches. In contrast, Bedwell’s ruler was universally applicable [page 199:] and used not inches but degrees as a standard measure of elevation.55 As with the carpenter’s rule, Bedwell was here diagnosing faults in contemporary craft practice and offering a mathematical resolution of them. The prime element in the reformation of errors was the replacement of familiar craft instruments with those newly devised by the mathematical practitioner.56
Bedwell presumed that the errors in craft practice arose from the mechanicians’ lack of arithmetic and geometry. He therefore did not expect such artificers to understand the construction of his new instruments: ‘it is enough for them to know that it is so, although they be altogether ignorant why it is so’.57 Bedwell claimed that this attribution of ignorance was no disparagement to artificers. In this he was more than a little disingenuous. At the very least, an aspiring artisan would have been sharply stung by Bedwell’s critical comment, since it provided the precise rationale for the existence and superior status of the mathematical practitioners.58
Bedwell’s manoeuvre against the common sort of artificer (if not necessarily the more able) reinforces scepticism about any overly narrow alignment of the interests of mathematical practitioners and craftsmen.59 We should not assume that the revaluation of the mechanical arts enforced a positive revaluation of all [page 200:] mechanicians.60 Newly engaged with affairs which had previously been the exclusive concern of mechanicians, practitioners such as Bedwell used the theme of craft errors as a powerful weapon with which to dislodge craft interests and justify their own claims.
What was the response of mechanicians to the cultural politics of the mathematical practitioners? We have no record of the views of the vast majority of artisans; however, a small but significant number of the most able and articulate shifted their allegiance and accepted the claims of the mathematical practitioners. We can see the implications of this new affiliation in the case of one Richard More who, in 1602, published a book on mensuration which attempted to mediate between existing vernacular texts of mathematics and craft practice.61
More accepted and promoted the programmatic rhetoric of the mathematical arts. Even in his title he adopted the familiar theme of common errors: The Carpenters Rule ... With a Detection of Sundrie great errors, generally committed by Carpenters and others in measuring of Timber. However, unlike Bedwell, More was no graduate but rather a craft member of the Carpenters’ Company.62 However, More’s authorities were no longer his brethren in the Carpenters’ Company; instead they were the mathematical authors to whom he was subordinate in learning and [page 201:] status.63 According to More’s recommendation, aspiring artificers should attend the recently founded Gresham College to directly meet their new masters.64
Thus More accepted the legitimacy of the mathematical practitioners’ position. Indeed his work is indirect evidence of the practitioners’ success in carving out a space for themselves. Bedwell, one of the architects of this success, was also ultimately a beneficiary, for More posthumously publicised Bedwell’s carpenter’s rule:
While this book was in printing I came to the sight of a ruler sometime invented by one Master Bedwell; which, as it is easy, so it is most speedy, and not less certain (being truly made), for the measuring of timber and board; which I expect and hope will be shortly published for the common good.65
Richard More was therefore a representative of those mechanicians who sought an alliance with the mathematical practitioners. The crucial point is that he did so on the practitioners’ terms: he stood to gain from the newly fashioned prestige of ‘the mathematicalls’ only by accepting the programme and values of the practitioners. More took up the task of preaching the mathematicalls and reforming craft errors from within.
Bedwell’s conception of the mathematical practitioner’s role thus had major implications for the status of mechanicians. Bedwell presented himself in a space between gentlemen and artificers, between patrons and craftsmen. In doing so he marked out the boundaries of his own independent cultural territory, characterising [page 202:] those mechanicians unable or unwilling to come to terms with the programme of the mathematicalls as inferior in status and authority. Such men were required to accept the guidance of the practitioner, just as those who did not understand why the practitioners’ instruments worked ‘must leave [the reason] unto the learned in the mathematical arts’.66
3. EXPERT ARTISANS AND INGENIOUS GENTLEMEN
Thomas Bedwell’s texts indicate the problematic nature of the relationship between practitioners and mechanicians: Bedwell offered mathematics as a beneficent helping hand but also suggested that mechanicians needed to move aside to accommodate the claims of the mathematical practitioner. Ultimately, Bedwell aspired to control the work of the mechanician.
However, aspiration rarely translates directly into achievement. While Richard More’s book indicates that the attempt to take over at least the mensurational component of craft practice was taken seriously, we cannot conclude that all mechanicians held the same positive views as More. I therefore want to begin this section with two of the remaining Dover participants for whom mathematical practice was more a useful resource than a manifesto. John Symonds and Paul Ive were both principally occupied in the world of construction and they enable us to explore the edges of mathematical practice, where its attractions dimmed and where it was not necessarily granted highest priority. Capturing the contours of mathematical practice means addressing these cultural borders as well as the central highlands. [page 203:]
John Symonds (or Symans, as he also signed himself) was a widely experienced craftsman and building consultant.67 He was considered capable in a range of materials, variously being responsible for masonry work and stone carving as well as latterly holding the official post of master plasterer. Moreover, by apprenticeship, he was a member of the Joiner’s Company. From his will of 1597 it is clear that he had practised successfully and had accumulated not only property but also the fine clothes which could mark him out as a man of substance.
From the late 1560s onwards, Symonds spent time as an officer in the royal works. But he also took on other work and seems to have had a special link with the Cecil family, producing plats and designs for both Lord Burghley and his son Robert Cecil.68 Moreover, though primarily involved in architectural construction and decorative work, he was also consulted on other technical matters. He advised on a planned masonry sluice for Dover harbour and served as Surveyor of Dover Castle. In a related military capacity, he also proposed a design for an earthen gun-platform.
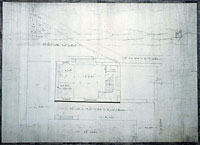
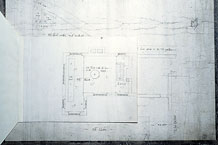
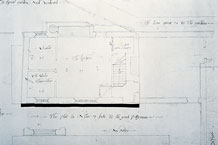
However Symonds gave no indication of a widely based commitment to the programme of the mathematical arts. Not that he was unaware of the familiar devices of mathematical practice. He was, for example, sophisticated in his preparation and use of plats. Several survive to record features of buildings and to propose designs. His plats are typically scaled and often make skilful use of flaps to indicate either alternatives, before-and-after schemes or the different storeys of single buildings (for [page 204:] an example, see Figure 4.3, Figure 4.4, Figure 4.5 and Figure 4.6).69 Moreover, in order to produce scaled plats, Symonds presumably did some surveying. This was not necessarily carried out only with cord and pole, for in his will he bequeathed instruments typical of mathematical practice. His brother received ‘my geometrical instrument of wood called Jacob’s staff’ while a former apprentice was rewarded with half of Symonds’ stoneworking tools, half of his plats, his best case of iron compasses, his finest gilded pencil and a ‘geometrical square of latten [brass] for measuring of land’.70
Symonds thus appears as an upwardly mobile craftsman for whom the title of architect would be neither foreign nor unwelcome. His carefully prepared plats and ownership of instruments show his familiarity with the resources typically employed by the mathematical practitioner. But Symonds does not appear as a zealous convert to the programme of the mathematical arts: unlike the practitioners already discussed in this and previous chapters, Symonds was not an author. While instruments and plats were important bearers of the claims and values of mathematical practice, texts (whether published or for private manuscript circulation) remained the dominant medium for its advocacy. Symonds’ mathematical engagement therefore appears relatively modest; despite borrowing eclectically from their resources, he did not contribute directly to the development of the mathematical arts, nor did he promulgate [page 205: Figure 4.3] [page 206: Figure 4.4] [page 207: Figure 4.5] [page 208: Figure 4.6] [page 209:] them through teaching.
By contrast, Paul Ive was an author. Yet, as with Symonds, only a limited sense of engagement with mathematical practice can be discerned in his career.71 Ive was the Privy Council’s principal consultant on fortifications towards the end of Elizabeth’s reign, working in England, Wales, Ireland, the Channel Islands and the Low Countries. Though he had first come to prominence at Dover in 1584, where he was called in to supervise work on two harbour groins, thereafter he was concerned exclusively with military architecture; in his will of 1604 he describes himself as ‘servant to the king for his fortifications’.72
Although Ive was occupied with a blur of official activity in the 1590s he was not concerned only with the here-and-now of military necessity. In 1589 he had published a translation of a French text providing Instructions for the Wars, along with a much briefer treatise on fortification which he had composed himself. In 1600, a year after the second edition of his own fortification text, he dedicated his manuscript translation of Simon Stevin’s manual on fortification to the Earl of Northumberland.73
Concentrating on Ive’s military (rather than literary) practice, it is clear that, like Symonds, plats played an important role in his work. However, although often [page 210:] scaled, his plats were usually prospect views rather than the precisely measured and drawn vertical plans which Symonds produced.74 Yet Ive did carry out measured surveys in the field, certainly with a cord and probably by instrument too.75 But his plats’ greater attention to pictorial detail (through colour and the rendering of local topography) may indicate a distance from the geometric style characteristic of Symonds’ productions.
But Ive was not an innocent in mathematics. He was clearly numerate: in his Practise of Fortification, the detailed prescription for setting out Italianate angle bastions required basic numerical skills and practical geometry. He also had a wider sense of the mathematical arts. For example, in the dedication to his translation of Stevin, Ive praised the Earl of Northumberland’s ‘good foundation ... in the mathematicks’. But although Ive was familiar with the idea of the mathematical arts, his professional concerns revolved principally around questions of fortification. It was in the military role of a designer and surveyor of fortifications that he specialised rather than in the general role of a mathematical practitioner. He therefore held no special brief for mathematics; he seems to have been willing to accept what he could make use of, but unconcerned when he considered another area of expertise to be of greater utility or priority. [page 211:]
Ive’s selective approach to competing skills is evident from an example in his Practise of Fortification. He noted that one particular difficulty would be better dealt with by a practised soldier rather than by a geometrician or a mason, who would each have insufficient military experience. However, this was not necessarily a blanket denunciation of mathematicians, or indeed of masons. In another section, when dealing with the foundations for stone walls, he specifically recommended that the advice of masons be heeded.
Figures such as Paul Ive and John Symonds achieved worldly success through a technical career. But their careers and aspirations give the lie to any interpretation which overemphasises the claims articulated by Thomas Bedwell. Bedwell hoped that as a mathematical practitioner he would achieve control over the work of mechanicians. But he could not realistically expect to have a John Symonds or a Paul Ive as a subordinate. As they became senior figures in the military and civil spheres of construction these designers and builders had increasing access to power. Entrusted with strategic and financial responsibilities, they were independent of the authority of practitioners such as Bedwell.
Moreover, they did not reject mathematics. On the contrary, for such socially mobile mechanicians as Symonds and Ive, mathematics was a repository of techniques which could be pragmatically picked and chosen. These Dover participants neither had nor needed the commitments and values that inspired and informed the work of Digges, Baker, Bedwell and Borough. The difference between these two mechanicians and the practitioners was that for the latter, mathematics was crucial to their identity and self-perception. [page 212:]
Yet the examples of Symonds and Ive should not lead us towards a sharp opposition between mathematically committed practitioners and more distant and pragmatic mechanicians. The case of Mathew Baker has already indicated the extent to which a mechanician might adopt and adapt the programme of the mathematicalls. Another of the Dover participants shows that Baker was not the only mechanician to incorporate mathematics at the centre of his professional role.
In certain respects, Robert Stickells is similar to John Symonds.76 At the time of his death in 1620, Stickells referred to himself as a mason. But stone was not his only medium: throughout his career he took on a wider range of activities and responsibilities. The first record of his work is at Dover, where he was acting as an engineering supervisor, floating rocks away from the harbour mouth and constructing jetties during 1584-5. There is little subsequent evidence of Stickells’ activity until he achieved a secure position as a clerk in the royal Office of Works in 1597-8. Although he spent 20 years as a salaried official at Richmond, Stickells was not confined to an obscure administrative role. Rather, there is evidence that, as well as serving as a designer of royal works, he also acted as an architectural consultant to various private individuals.
In addition to Stickells’ surviving designs and his architectural advice, there are also several documents and letters which indicate his wider ambitions. These texts were written in the last few years of Elizabeth’s reign as part of Stickells’ attempts to secure patronage. They therefore give an important indication of the grounds on which Stickells thought himself most likely to be preferred. [page 213:]
Writing to seek the support of Robert Cecil, Stickells was careful to set himself up as much more than just a rude mechanical. Rather than a mere workman, he presented himself as an intellectual, for he acknowledged that ‘some suspend their judgement on me, saying that to study for the truth in that which I profess is but idleness and a vain mind in me’.77 A subsequent letter to Cecil, again seeking preferment, makes clear the range of Stickells’ studies.78 He diagnosed contemporary errors in a range of practices from house building and fortification to shipbuilding and gunnery. Errors arise because ‘all those works that heretofore hath been done and is daily done is imperfect and unjust, both in measure and in proportion’. Stickells explained where the fault lay: ‘the learned have but the theoric and not the practic’, while the workmen are ‘blind for want of further knowledge’:
how simple are those men that work daily and know not what a symmetry is, no not so much as to double his two foot rule that he carrieth up and down in his hand.
In contrast to such characters, Stickells sought to demonstrate his abilities by listing his devices and conclusions, and posing specific questions. Two short papers survive; one asks how the dimensions of buildings and ships are to be derived from each other by proportional rules, while the other summarises the same set of questions and also cites three of Stickells’ inventions for ships.79 The specific questions of these papers were intended as public challenges, ‘propounded unto the learned and skilful’, by which Stickells could exhibit his skill.80 [page 214:]
For Stickells, the expertise that enabled him to transcend the limitations of contemporary workmen lay in the mathematical arts. His references to measure and proportion are suggestive, especially his reiterated requirement that rules should generate dimensions for structures that are in just proportion, neither too big nor too little. Equally indicative is his claim to competence in practices such as fortification and artillery. But he is also more explicit: ‘my desire is that I might be put unto my trial, either in the mathematical sciences or in the rules of architecture, the which I profess’.81 Stickells thus built his claims for patronage on the basis of the mathematical arts. Indeed, he went further, for he also incorporated mathematical elements into his aesthetic notions.
There are two sorts of buildings, the one in sense, the other without sense. The antiques in sense, the modern without sense, because it is from circular demonstration without sense, for that no circle riseth in evenness of number. The antiques always in evenness of number because they are derived from an ichnographical ground .... There is no more but right and wrong in all things whatsoever, the square right, the circle wrong, etc.82
Although difficult to interpret, Stickells was apparently using the incommensurability of the straight and the circular to distinguish between ancient and modern architecture. What is important in the present context is that Stickells used the mathematical arts not just as an instrumental resource but as a significant feature of the identity which he presented to a patron. The idea of the mathematical arts was employed to help in distinguishing him from ordinary workmen, to expose contemporary errors and to establish himself as competent in all matters of properly proportional building. Stickells has a place with both Mathew Baker and the carpenter Richard More (Bedwell’s posthumous advocate) as a mechanician who rested his credentials on [page 215:] expertise in the mathematical sciences.
After Symonds, Ive and Stickells - a trio of architects and military engineers - I want to conclude this chapter with a figure who takes us into the wider world of mathematical practice. The use of Dover harbour as a principle of selection means that my cast of characters has inevitably been skewed towards construction. But of course, there are a number of contemporary mathematical practitioners who have no link with building activity, let alone the specifics of harbour design. It is therefore appropriate to end with a final Dover participant, John Hill, whose responsibilities lay in the area of valuations and surveying rather than construction.
Hill was usually described as an auditor, though we presently know little of his career. In 1584 and 1585 he regularly informed Principal Secretary Francis Walsingham of the progress of the works at Dover and he continued to figure in reports and agendas into the 1590s, when he seems to have been responsible for plats as well as instructions and accounts (see chapter 5). Yet although his career is obscure, Hill was not unknown to his contemporaries. Even before his supervisory service at Dover he had already been cited in print as an expert in what Edward Worsop took to be the three departments of surveying: mathematical, legal and judicial. Worsop went on to personally vouch that ‘M. John Hils an auditor understandeth arithmetic, geometry and perspective both speculatively and practically singularly well’.83
Hill was also in contact with other mathematical practitioners. When [page 216:] describing his ‘familiar staff’, John Blagrave noted that ‘the type of graduating this instrument commended by the author to the ingenious mathematical-minded gentleman Master Auditor Hill’.84 Hill’s connections can be pursued further for he was overseer to the will of the clockmaker Bartholomew Newsam. For his pains in ensuring that Newsam’s lands were sold for a fair price Hill was bequeathed ‘one crystal jewel with a watch in it garnished with gold and a great dial in a great box of ivory with two and thirty points of the compass’.85
What is the significance of these few surviving details of Hill’s acquaintances and skills? John Hill was evidently one of those ‘well-wishers to the mathematicks’ who are familiar from title pages and dedications. He was probably well-read and apparently experienced in the practice of surveying. It certainly seems clear that he was known as an aficionado of mathematical instruments. However, he could not be plausibly represented as a central figure in the contemporary mathematical arts, for he was never a prominent practitioner, responsible for publications or prestigious achievements. Hill’s significance stems precisely from his position on the fringes of mathematical practice. His career suggests that mathematical interests were not restricted to just a few familiar names, but that there existed an informed audience for whom the texts and instruments of the mathematical practitioners were of genuine importance. As with any social or intellectual programme, the development of mathematical practice depended on its movement out beyond a small circle of early advocates. Moreover, as a phenomenon of the printed book, mathematical practice [page 217:] did not just depend on the patronage of great magnates; it also engaged a public made up of readers of the middling sort. John Hill is suggestive of the largely anonymous majority whose personal support (and purchasing power in the market) was essential to the success of the mathematical practitioners.
FOOTNOTES
1. The monumental work of David W. Waters, The Art of Navigation in England in Elizabethan and Early Stuart Times (London, 1958) remains unsurpassed in this respect. The flavour of Waters’ work is conveniently captured in a more recent overview: ‘English navigational books, charts and globes printed down to 1600’, Revista da Universidade de Coimbra, 33 (1985), 239-257.
2. Alae seu Scalae Mathematicae (London, 1573), sig. I3v-4r. Note that Pantometria (London, 1571) also promised a future treatise on cosmography and navigation (final page of book 1, ‘Longimetra’). However, it seems more likely that this promise was retained in Leonard Digges’s original manuscript by Thomas, rather than being newly introduced by him.
3. David Waters, ‘Columbus’s Portuguese inheritance’, Mariner’s Mirror, 78 (1992), 385-405, pp. 397-9.
4. Digges’s most likely source for Cabot’s presumption was Richard Eden’s anecdote in the preface to his translation of Martin Cortes’s Art of Navigation (London, 1561).
5. Unfortunately, there is insufficient space here to present a detailed reconstruction of Digges’s hypothesis. In the brevity of modern notation, it amounts to the relationship tan υ = tan λ.sin θ, where υ is the variation, λ the longitude and θ the latitude. A principal feature of the model is that variation is zero at all points on the equator (except due east and west where it is undefined because the magnetic and horizontal planes coincide). According to the model, variation increases with latitude; note in particular that υ → λ as θ → 90°. (The variation is not defined at the pole itself since longitude has no meaning there.)
6. The basic geometrical configuration of a dial of arbitrary declination and inclination does indeed closely match the geometry of Digges’s magnetic hypothesis. But Digges seems to slip up in stating that the variation is ‘always exactly equal to the angle contained of the meridian line and line of the style’. For, in the dial’s configuration, the angle between the horizontal meridian line and the line of the style is simply the dial’s latitude, a constant independent of the dial’s inclination. Nevertheless relationships similar to the equation governing Digges’s magnetic model can be determined. We can take as an example the case of a vertical declining dial (since a dial which both inclines and declines is equivalent to a vertical declining dial made for a different latitude). Digges matched magnetic longitude to the dial’s declination and the needle’s latitude to the complement of the dial’s inclination. So if δ is the declination (measuring south to east) and α is the dial’s inclination (angle between style and vertical noon-line), then tanβ = tan(90-α).secδ, where β is the angle between the sub-style and the foot of the wall. Alternatively, sinγ = cos(90-α).sinδ, where γ is the angle between the style and the sub-style.
7. Digges is thus an early and highly important exception to the general characterisation of mathematical practitioners and their work on magnetism given by J.A. Bennett, ‘The mechanics’ philosophy and the mechanical philosophy’, History of Science, 24 (1986), 1-27, especially pp. 7 and 12. However, the point is not that the generalisation is wrong, but rather that at this early stage in his career Digges does not fit comfortably within the category of mathematical practitioner. We shall see below that in later life he significantly revised his characterisation of proper navigational practice.
8. Leonard and Thomas Digges, Stratioticos (London, 1579), sig. A4r-v.
9. New Attractive, sig. B1v. I retain Norman’s spelling of ‘conceipt’ because neither of the modern terms ‘concept’ or ‘conceit’ adequately captures its connotation.
10. In the dedication, Norman specifically cited the tales of Archimedes’s Eureka moment and the celebrations attending the discovery of the Pythagorean theorem (sig. A2r-v). These were witnesses to the ‘incredible delight’ of new devices and inventions which Norman had now also experienced.
11. Waters (footnote 1), p. 79 n.1. For other evidence of Aborough’s navigational work, see David Starkey (ed.), Henry VIII. A European Court in England (London, 1991), p. 149. Aborough was also involved in harbour proposals; indeed, by striking coincidence, he worked at Dover harbour (among other places) during Henry VIII’s reign: H.M. Colvin (ed.), History of the King’s Works, 6 volumes (London, 1963-82), IV, pp. 454, 747, 751. The disparity in surname between John and William is not necessarily significant: William was often rendered as ‘a Borough’ or ‘Aborough’: Anthony Jenkinson to William Cecil, 26 June 1566, in E. Delmar Morgan and C.H. Coote (eds), Early Voyages to Russia and Persia, Hakluyt Society, 1st series, 72-3 (London, 1886), II, p. 188; endorsement on a letter from Borough to Francis Walsingham, 14 January 1578, SP12/129/11; J.R. Dasent (ed.), Acts of the Privy Council, XIII, p. 81 (14 June 1581); SP12/159/52 (summer 1581); and ‘The Observations of Sir Richard Hawkins knight in his Voyage into the South Sea’ (1593), in Clements R. Markham (ed.), The Hawkins’ Voyages, Hakluyt Society, 1st series, 57 (London, 1878), p. 183.
12. On Stephen Borough, see Waters (footnote 1), pp. 103-7.
13. Richard Hakluyt, The Principal Navigations, Voyages and Traffiques of the English Nation, 12 vols (Glasgow, 1903-5), III, p. 210.
14. See SP12/243/110 for his discussion of ship design and the calculation of tonnage, topics which he must have discussed with the royal master shipwright Mathew Baker (chapter 3 above).
15. Borough’s administrative responsibilities and his actions at sea are too numerous to list here. His entry in the Dictionary of National Biography gives some examples.
16. G. G. Harris, The Trinity House of Deptford, 1514-1660 (London, 1969), p. 273.
17. Will made 26 July 1598 and proved 28 November 1598 (PRO PCC 89 Lewyn; PROB 11/92, ff. 229r-230r).
18. The letter was printed in the second edition (1598-1600) of Hakluyt (footnote 13), III, 209-212. Borough referred to the accompanying map in the Discourse, sig. F3v, and dated it there to 1578.
19. 1578 letter (footnote 18).
20. Three years after his 1576 discussion, Digges again promised to publish on navigation; Stratioticos (1579), sig. a4r. Norman was to take the same swipe in his later comments: ‘I would wish the learned to use modesty in publishing their conceits and not disdainfully to condemn men that will search out the secrets of their arts and professions ... No more than they would that others should judge of them for promising much and performing little or nothing at all’, New Attractive, sig. B2r.
21. The commendable if impractical methods of ingenious mathematicians are discussed in William Gilbert, On the Magnet, translated by Silvanus P. Thompson et al. (1900; republished, New York, 1958), book IV, chapter 12 (‘On finding the amount of variation...’), p. 172. The details strongly suggest that Borough rather than, say, Edward Wright was the object of these comments, which label as excessively exotic just those sophisticated techniques that were described by Borough. That Wright was not the target is also suggested by Mark Ridley’s later claim that this chapter was not written by Gilbert himself but actually by Wright: Magneticall Animadversions (London, 1617), pp. 9-10.
22. Discourse, sig. G2v. On Coignet, see Ad Meskens, ‘Michiel Coignet’s nautical Instruction’, Mariner’s Mirror, 78 (1992), 257-76. For Medina, Waters (footnote 1).
23. Discourse, chapter 10. Norman also tackled the same topic in chapter 10 of The New Attractive.
24. Discourse, chapter 9. Borough offered his calculation primarily as a mathematical exercise and refrained from attributing physical significance to the determined point. In chapter 12, he remarked that additional observations of the dip other than those already made at London were necessary before anything further could be inferred about Norman’s ‘point respective’.
25. In modern notation, the polar model generates the following relationship 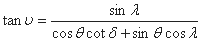
where υ = variation, λ = longitude, θ = latitude and δ = distance of magnetic pole from geographic pole. Note that, using the London variation of 11¼° east to generate parameters, Digges’s hypothesis gives a theoretical value for the variation at Vaigatz of 71° 14’ east, even further from Borough’s observed value than the prediction of the polar model. This may shed extra light on Digges’s dismissal of mariners’ variation determinations!
26. Discourse, sig. G3v. This has been taken to represent an explicitly natural philosophical ambition: Bennett (footnote 7), p. 16. But Borough’s language of a hypothesis to save the irregular phenomena of variation refers not to natural philosophy but to mathematical astronomy. And this is the dominant literary and explanatory model for the Discourse as a whole. Of course, the objectives of mathematical astronomy were themselves undergoing revision at this time, as witnessed by the astronomical work of Digges. But Borough’s principal hope was that the variation ‘may be reduced into method and rule’.
27. Discourse, sig. G3v. For an example of the data received by Borough, see Hakluyt (footnote 13), III, 214-47. This is a report of a journey into Persia undertaken during 1579-81 by Borough’s nephew Christopher Borough. Assembled from Christopher’s letters to his uncle it contains several variation observations: see pp. 220, 229, 235. Borough also encouraged the cartographic representation of variation results. He prepared a chart of the North Atlantic (dated 1 June 1576) on which were recorded observations of variation during Frobisher’s first voyage (Hatfield House, CPM I, 69). For reproductions and discussion of this chart, see R.A. Skelton and John Summerson, A Description of Maps and Architectural Drawings in the Collection made by William Cecil First Baron Burghley now at Hatfield House (Oxford, 1971), catalogue no.122 and Waters (footnote 1), pl. xxxvi, pp. 144-6, 528-9. For examples of land-based variation observations in northern Russia that Borough was himself party to, see Stephen Borough’s journals of the voyages of 1556 and 1557 (Hakluyt, II, 322-44 and 363-75, pp. 334, 336, 339, 363, 364, 367).
28. Discourse, sig. *4r-v. David C. Goodman, Power and Penury. Government, Technology and Science in Philip II’s Spain (Cambridge, 1988), chapter 2 cites important evidence of structural problems in the teaching and supervisory functions of the Casa de la Contratación.
29. BL Lansd. MS 10, f. 133r; written in August 1568 for a proposed voyage beyond Vaigatz towards Cathay by James Bassendine, James Woodcock and Richard Brown on behalf of the Muscovy Company. A slightly expanded version appeared anonymously in Hakluyt (footnote 13), III, 122-4. Borough later supplied instructions for Pet and Jackman’s 1580 voyage towards the same destination; Hakluyt, III, 259-262. A third, anonymous set of instructions for Thomas Bavin in 1582 may also be by Borough; printed in Waters (footnote 1), appendix 14. It was certainly prepared by someone very closely familiar with Borough’s Discourse. For a discussion, see E.G.R. Taylor, ‘Instructions to a colonial surveyor in 1582’, Mariner’s Mirror, 37 (1951), 48-62. Note that Borough had himself been the recipient of instructions earlier in his career. Sebastian Cabot’s ordinances for the 1553 voyage laid down that the youths on board were to receive tuition in navigation; Hakluyt, II, 195-205, item 15 (p. 199). Cabot also stipulated procedures for observations and record-keeping, see item 7 (p. 197). Both Stephen and William Borough were also in contact with John Dee at this time: Waters (footnote 1), p. 210n.
30. Elizabeth Story Donno (ed.), An Elizabethan in 1582. The Diary of Richard Madox, Fellow of All Souls, Hakluyt Society, 2nd series, 147 (London, 1976), p. 224.
31. For Harriot’s Virginia voyage, see John W. Shirley, Thomas Harriot. A Biography (Oxford, 1983), ch. 4 and, for his navigational work, including his studies of magnetic variation: Jon Pepper, ‘Harriot’s earlier work on mathematical navigation: theory and practice’, in John W. Shirley (ed.), Thomas Harriot: Renaissance Scientist (Oxford, 1974), 54-90. Wright’s narrative account of his 1589 voyage to the Azores in the Earl of Cumberland’s fleet is appended to his Certaine Errors in Navigation (London, 1599) while his variation observations appear in the book itself at sigs M4v-N1r.
32. Note that, in studying the contending pronouncements of Digges, Norman and Borough, I have sought to examine the formation of the mathematical practitioner’s identity, rather than attempt a comprehensive account of magnetic variation. A fuller story of English magnetic study would have to include figures such as John Dee; see E.G.R. Taylor, ‘John Dee and the nautical triangle, 1575’, Journal of the Institute of Navigation, 8 (1955), 318-25.
33. For the importance of instruments see, for example, J.A. Bennett, The Divided Circle. A History of Instruments for Astronomy, Navigation and Surveying (Oxford, 1987), A.W. Richeson, English Land- Measuring to 1800: Instruments and Practices (Cambridge, Mass., 1966) and E.G.R. Taylor and M.W. Richey, The Geometrical Seaman (London, 1962). Note that references to instruments take up 10 columns in the index to Waters (footnote 1).
34. G. L’E. Turner, ‘Mathematical instrument-making in London in the sixteenth century’, in Sarah Tyacke (ed.), English Map-Making, 1500-1650, (London, 1983), 93-106 and Joyce Brown, Mathematical Instrument-Makers in the Grocers’ Company 1688-1800, with notes on some earlier makers (London, 1979). The career of Thomas Hood in the 1590s suggests both that there was cooperation between practitioners and makers and also that the boundaries between these two categories were fluid: Stephen Johnston, ‘Mathematical practitioners and instruments in Elizabethan England’, Annals of Science, 48 (1991), 319-344, pp. 337-40.
35. My account of Bedwell is adapted from the longer version in Johnston (footnote 34), pp. 320-330. For the detailed evidence of Bedwell’s Cambridge career and other points in this paragraph, see p. 321.
36. Bedwell’s first involvement with this protracted project was probably in April 1582 (PRO SP12/153/27-29). He was still offering suggestions and estimates in March 1583 (PRO SP12/159/9). See chapter 5 for further details of Bedwell’s Dover work.
37. Burghley to Walsingham, 12 August 1582, PRO SP12/155/14.
38. Simon Adams kindly provided me with transcripts of the Earl of Leicester’s household accounts for 1584-5 prior to their publication in the Camden Society series.
39. Calendar of State Paper, Foreign, June 1586 to March 1587 (London, 1927), p. 319. He is presumably the ‘beduwel’ listed in the Dutch records as a follower of Leicester; see R. Strong and J.A. van Dorsten, Leicester’s Triumph, Publications of the Sir Thomas Browne Institute, special series, 2 (Leiden, 1964), p. 110. For the duties of the pioneers see C.G. Cruickshank, Elizabeth’s Army, second edition (Oxford, 1966), p. 25. Recall that Thomas Digges also took part in this military venture.
40. PRO SP12/199/22; Henry Radcliffe, 4th Earl of Sussex to Lord Burghley, from Portsmouth, 10 March 1586/7.
41. Colvin (footnote 11), IV, p. 604.
42. Bedwell was buried on 30 April 1595, see M.S.R., Notes and Queries, 2nd series, 10 (1860), 74-5.
43. For William Bedwell’s publications on Thomas’ carpenter’s rule, see Eileen Harris, British Architectural Books and Writers 1556-1785 (Cambridge, 1990).
44. I know of no surviving examples of either rule. The brief manuscript tract on the carpenter’s rule is Bodleian Tanner 298 (4) (referred to hereafter as Tanner); it is bound with several printed works. The text on the gunner’s rule is Bodleian MS Laud 618 (hereafter referred to as Laud).
45. MS Laud f. 1r.
46. For the diffuse but recognisable role of the military engineer, see Colvin (footnote 11), IV, pp. 409-14.
47. PRO SP12/153/27.
48. The following account is based on the introductory sections of the tract on the gunner’s rule.
49. See the dedication to his Nova Scientia, in Stillman Drake and I.E. Drabkin (eds), Mechanics in Sixteenth-Century Italy (Madison, 1969), p. 65. For a general account of gunnery in this period, see A.R. Hall, Ballistics in the Seventeenth Century (Cambridge, 1952).
50. Bedwell’s patent as storekeeper is dated 15 January 1589, see O.F.G. Hogg, The Royal Arsenal, 2 volumes (London, 1963), I, p. 169 n. 111.
51. BL Sloane MS 871, f. 150r-v.
52. Warwick to Burghley, 17 January 1588/9, BL Lansd. 59/5.
53. Laud f. 1v. It is unclear whether Bedwell’s tract was itself used to gain patronage, or whether it merely represents arguments that he had already put forward. The text was certainly written about the time of his appointment in January 1589, but whether just before or after cannot be confidently determined. Bedwell states that it was two years since he had returned from the Netherlands and it is clear from the Earl of Sussex’s letter (footnote 40) that he was back in England by March 1587. The issue is complicated by the Earl of Warwick’s obscure hint that Bedwell may have been marked out for the post of storekeeper a good deal earlier than his actual appointment (footnote 52).
54. Tanner, f. 2r.
55. Laud f. 3r-v. The opposition between alternative units in gunnery parallels the contemporary contest between linear and angular measure in surveying; J.A. Bennett, ‘Geometry and surveying in early seventeenth-century England’, Annals of Science, 48 (1991), 345-54, p. 346.
56. For a craft example of a (ship?) carpenter’s rule, as well as other mathematical versions, see Stephen Johnston, ‘The carpenter’s rule: instruments, practitioners and artisans in 16th-century England’, to appear in G. Dragoni, A. McConnell and G.L’E. Turner (eds), Proceedings of the XIth International Scientific Instrument Symposium, Bologna, September 1991 (Bologna, 1994).
57. Tanner, f. 1r.
58. Compare Robert Norman’s response to Thomas Digges’s similar remarks, as discussed in the preceding section.
59. As suggested by, for example, Christopher Hill, Intellectual Origins of the English Revolution, corrected edition (Oxford, 1980), ch. 2, ‘London Science and Medicine’.
60. Paolo Rossi, Philosophy, Technology and the Arts in the Early Modern Era, translated by S. Attanasio (New York, 1970), pp. 29-30. Rossi distinguishes the general process of the revaluation of the mechanical arts from the particular changes in the status of mechanicians. Rossi’s stress on upward mobility and harmonious cooperation between artisans and intellectuals should be qualified by a more extensive consideration of strategies such as Bedwell’s.
61. More’s text thus has a similar character to Edward Worsop’s earlier work on surveying, A Discoverie of Sundrie Errours and Faults Daily Committed by Landmeaters, Ignorant of Arithmetike and Geometrie (London, 1582).
62. More identifies himself as a carpenter on his title page. For his membership of the company see A.M. Millard, Records of the Worshipful Company of Carpenters, VII, Wardens’ Account Book 1592-1614 (London, 1968), where he is indexed as Richard Moore.
63. See his citation of authors in The Carpenters Rule (London, 1602), p. 56.
64. Ibid., sig. A4v.
65. Ibid. Bedwell’s ruler continued to be used, even before it was advertised and described in print by his nephew William Bedwell. It was probably part of the instrumental repertoire of contemporary mathematicians. Certainly, Henry Briggs was offering instruction on its use in 1607: Briggs to Ralph Clarke in Stephen Jordan Rigaud (ed.), Correspondence of Scientific Men of the Seventeenth Century, 2 vols (Oxford, 1841), I, pp. 1-3.
66. Tanner, f. 1v.
67. For details of Symonds’ career, see John Summerson, ‘Three Elizabethan architects’, Bulletin of the John Rylands Library, 40 (1957), 202-228, with a few extra points in Colvin (footnote 11), III and IV.
68. In addition to the sources already cited, John Summerson, ‘The building of Theobalds, 1564-1585’, Archaeologia, 97 (2nd series 47) (1959), 107-126.
69. For other examples of Symonds’ plan views of buildings, see Hatfield House, CPM I, 10 and 19 (Holy Trinity church), CPM II, 9 and 10 (‘Chelsea Great House’) and CPM II, 21 & 21a (Havering); these plats are all described in Summerson and Skelton (footnote 27). Hatfield House CP 143/47 is a simpler drawing of a gateway for Theobalds, reproduced as plate XXVIIc in Summerson (footnote 68). PRO MPF 122 is a plat of Dover harbour by Symonds. For more on plats with flaps, see chapter 5.
70. Cited in Summerson (footnote 67), appendix 1. Note that some of Symonds’ professional associates also had brass instruments. Symonds first appears in 1566-7 when he was working as a draughtsman under the surveyor of Portsmouth’s fortifications, Richard Popinjay. In an inventory taken after his death in 1595, Popinjay’s study was found to contain ‘a small instrument of brass’ and ‘great brass instrument’; Colvin (footnote 11), IV, p. 524 n. 3.
71. There is no full and accurate biography of Ive available. Partial accounts can be found in the Dictionary of National Biography, in Rolf Loeber, ‘Biographical Dictionary of Engineers in Ireland, 1600-1730’, The Irish Sword, 13 (1977-9), 30-44, 106-22, 230-255, 283-314, p. 240f and in Martin Biddle’s introduction to the 1972 facsimile of Ive’s Practise of Fortification (1589). Many of the details of his career can be located through the indexes to Colvin (footnote 11), III and IV.
72. PRO PCC 9 Hayes (PROB 11/105, f. 67r-v), made 24 May 1604, proved 21 February 1604/5. Ive also referred to himself as ‘gent’.
73. Petworth House Archives (West Sussex Record Office) MS HMC 138. There is a 1604 copy of this translation (omitting Ive’s name) in Trinity College, Cambridge: The Principal Works of Simon Stevin, 5 volumes, vol. 4: The Art of War, edited by W.H. Schukking (Amsterdam, 1964), pp. 34-6.
74. For example, BL Cott.Aug. I.i.30 (Elizabeth Castle, Jersey); and Hatfield House CPM II,51 (Milford Haven). This latter attribution is not noted by Summerson and Skelton (footnote 27). I have not attempted a full catalogue of plats by or attributed to Ive. Such a listing would be technically difficult for there exist numerous anonymous plats of fortifications from Ive’s Irish campaign and these would all have to be examined. His work is also widely spread. There are examples of plats or drawings either signed by him or attributed to him in the British Library, Trinity College (Dublin), Hatfield House, Petworth House, Lambeth Palace Library, the National Maritime Museum and the National Library of Ireland.
75. In his ‘brief description of Milford Haven’, George Owen noted that Ive ‘liked well’ of a certain piece of ground ‘and measured it with his cord’; Henry Owen (ed.), The Description of Penbrokeshire [sic], Cymmrodorion Record Series, number 1, 4 parts (1892-1936), part 2, p. 549. It seems likely that Ive could have surveyed the large expanse of this natural harbour and mapped it to scale only with the aid of instruments, rather than just a cord.
76. I have taken biographical information on Stickells from Summerson (footnote 67) with some additional details from Colvin (footnote 11), IV. On the basis of the material published by Summerson, Stickells also appears in Frances Yates, Theatre of the World (London, 1969), pp. 106-7.
77. Hatfield House, Cecil Papers 90/168.
78. Hatfield House, Cecil Papers, 81/50, 20 August 1600.
79. Printed from BL Lansd. 84/10.i and ii in Summerson (footnote 67), appendix 3. Summerson’s transcription is generally accurate though he omits a line in the second document. Note that Stickells’ inventions were not just paper devices. Summerson notes that in 1595 Stickells constructed a small pinnace which could be dismantled, transported on a wheeled sled, and then reassembled for launching.
80. Hatfield, CP 81/50: ‘I published my propositions unto the view of the world and hath been forth near this two years, both unto the learned and the skilful, and yet unanswered or unperformed either of aliens or of English men’. It is not clear whether Stickells actually had his ‘propositions’ printed, or whether they were circulated in manuscript. There is no record of any relevant entry in STC, either under his name or anonymously.
81. Hatfield, CP 81/50.
82. Summerson (footnote 67), p. 228.
83. A Discoverie of Sundrie Errours (London, 1582), sig. K1v. Perspective here means the graphic rendering of ‘prospects’ rather than either painterly perspective or formal optics, cf. sig. J3v.
84. Baculum Familiare (London, 1590), p. 13.
85. PRO PCC 86 Nevell (PROB 11/82), made 7 January 1586 and proved 18 December 1593. For Newsam as a clockmaker, see Cedric Jagger, Royal Clocks (London, 1983), pp. 13-15, 309. In addition to the sundials mentioned in Newsam’s will, there is also a case of brass drawing instruments signed with his name in the British Museum; see F.A.B. Ward, A Catalogue of European Scientific Instruments in the Department of Medieval and Later Antiquities of the British Museum (London, 1981), p. 86.